As a small business owner focused on success, you may reach a point where you’re looking at acquiring new equipment or assets to help grow your company. But how do you know if investing in the equipment or asset is worth it and that you’ll get a positive return on your investment?
A net present value (NPV) calculation, also known as an npv calculation can help you make your decision. What does npv tell you? The net present value looks at the future cash flow that an asset—in this case, the equipment you want to purchase—is going to generate and discounts it to show the present value. After these discounted cash flows are added up, you then subtract the amount of the initial investment, or the cost of the asset.
If the net present value is positive (greater than 0), this means the investment is favorable and may give you a return on your investment. If it’s negative, you may end up losing money over the course of the project.
So how do you calculate npv?

Calculate Net Present Value (NPV): Which Formula Should You Use?
There are two formulas to calculate the net present value. Which formula you will use depends on whether the projected cash flows generated by the asset are the same amount each year or if they’re different amounts.
If the asset is estimated to generate the same amount of cash flow each year, you would use this formula:
R x ((1-(1+i)-n)/i) – Initial Investment
- The R represents the net cash flow for each time period.
- The i is the discount rate that will be used to find the present value of the future cash flows. The discount rate can also be thought of as the desired rate of return you could get on your investment.
- The n represents the time period you’re looking at to value the asset.
If the projected cash flows are a different amount for each year, you would use a different formula:
(C for Period 1 / (1+r)1) + (C for Period 2 / (1+r)2 + … C for given time period t / (1+r)t) – Initial Investment
In this formula, the C represents the cash flow for the given time period. The r represents the discount rate, or your desired rate of return. The t represents the time period. In this formula, you’re discounting each projected cash flow to find the present value. You then add the discounted cash flows together and subtract the cost of the initial investment from that sum. The difference is the net present value.
An Example
Let’s revisit the example from the top of the story. The piece of equipment you’re looking to purchase is going to cost you $25,000. Over the next five years, the equipment is estimated to generate an additional $6,000 a year. The discount rate you’ve chosen is 5%. Is it worth the investment?
Because the projected cash flows are the same for each of the five years, you would use the first formula. Here’s what it looks like:
| Formula | R x ((1-(1+i)-n)/i) – Initial Investment | |
| Plug in the information | $6,000 x ((1-(1+0.05)-5)/0.05) – $25,000 | |
| Net present value (NPV) | $6,000 x ((1-(1.05)-5)/0.05) – $25,000 | = $976.86 |
If the equipment is estimated to generate different cash flows for each year, you would use the second formula to find the net present value. Say the equipment is going to generate an additional $5,000 in the first year, $5,500 in the second year, $3,000 in the third year, $2,000 in the fourth year, and $1,500 in the fifth year. The rest of the scenario—initial cost of investment and discount rate—remains the same.
| Formula | (C for Period 1 / (1+r)1) + (C for Period 2 / (1+r)2 + … C for given time period t / (1+r)t) – Initial Investment | |
| Cash Flow for Year 1 | $5,000 / (1+0.05)1 | = $4,761.90 |
| Cash Flow for Year 2 | $5,500 / (1+0.05)2 | = $4,988.66 |
| Cash Flow for Year 3 | $3,000 / (1+0.05)3 | = $2,591.51 |
| Cash Flow for Year 4 | $2,000 / (1+0.05)4 | = $1,645.40 |
| Cash Flow for Year 5 | $1,500 / (1+0.05)5 | = $1,175.29 |
| Net Present Value | ($4,761.90 + $4,988.66 + $2,591.51 + $1,645.40 + $1,175.29) – $25,000 | = -$9,837.24 |
Over the next five years, the equipment is estimated to generate $30,000. The present value of the $30,000 is about $25,976.86. This means the money the equipment is generating for your business over the next five years is worth more than the initial investment of $25,000—about $976.86 more. So you may see a positive return on your investment.
In this example, the equipment is expected to generate an additional $17,000 over the next five years. After discounting the projected cash flow, that money is worth about $15,162.76 in present time. This is $9.837.24 less than the $25,000 initial investment—represented by a negative net present value. Because of this, the equipment may not provide a positive return on your investment and you may instead experience a loss.

Finding Net Present Value (NPV) Equation in Excel
The Excel spreadsheet software includes a net present value function. This allows you to quickly discount the projected cash flows and find the present value. Check out this video to follow along.
To find the net present value using Excel, you’ll need to set up your spreadsheet properly. Create a column for “Period,” and put in 0 through the number of years you’re looking at. Label the next column “Cash Flow” and put in the corresponding numbers for each time period. For Year 0, you’ll put in the initial investment cost (represented by a negative number).
The net present value function is “=npv(rate, value 1, value 2, ….).” In a separate cell, start putting in the function. You can click on each cell as you fill in the formula or manually type in each cell number. When you press enter, you’ll find the present value of the projected cash flows.
To find the net present value, you’ll have to go back into the Excel function. You can double-click the cell where you completed the function earlier. At the end of the function, put in an addition symbol and the cell number where your initial investment cost is. This tells Excel to find the present value of the cash flows and then add in the initial cost of the investment. Because it’s a negative number, the initial investment will be subtracted from the present value cash flows.
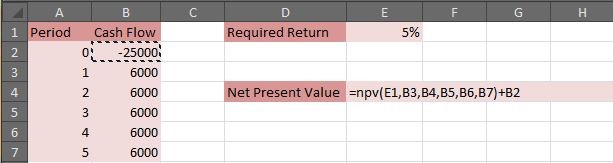
This is what the net present value function looks like while you’re filling in the formula with data from the Excel sheet.
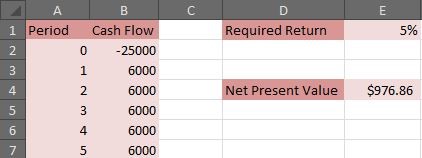
In this example, the projected cash flows were even throughout the five years. Using the function in Excel, the net present value is $976.86.
You can perform a similar calculation for the second example, where the cash flows are different for each year.
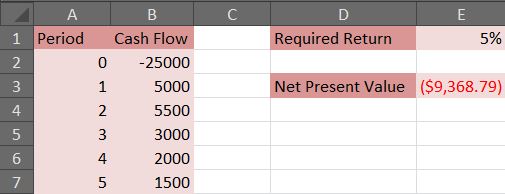
In this case, Excel calculates the net present value of -$9,837.23, which means the asset is likely to lose you money over the five years.
Positive Net Present Value (NPV)
When you calculate the net present value of an asset, you’ll get either a positive or negative number. It’s this number that will help you make a more informed decision on whether to invest in the asset.
A positive net present value means you may get a return on your investment. It shows you that while you are losing money up front (for the initial investment), the asset is going to generate cash flows in the future that in total are worth more than the initial cost. It’s important to remember that there are limitations with the net present value (NPV) calculation. Since it’s based off of assumptions of projected cash flow, the calculation is only as good as the data you put into it.
If your NPV calculation results in a positive net present value, it means this could be a good investment opportunity.
Negative Net Present Value (NPV)
If your NPV calculation results in a negative net present value, this means the money generated in the future isn’t worth more than the initial investment cost.
A negative net present value means this may not be a great investment opportunity because you might not make a return. Essentially, a negative net present value is telling you that, based on the projected cash flows, the asset may cause you to lose money. There are other factors outside of the net present value calculation that could still make this a potentially good investment, such as providing enhanced safety or increasing company morale.

Present Value and Net Present Value (NPV)
Because money is worth more today than it is tomorrow, you need to find out how much future projected cash flows are worth in today’s time—or present value. The present value is the part of the net present value formula where projected cash flows for each year are discounted by a certain rate.
The net present cash flow is the sum of the discounted cash flows (after finding the present value of the projected cash flows), from which you will subtract the initial cost of investment. The resulting net present value will tell you whether you can expect to get a positive or a negative return on your investment, based on looking at the asset’s projected cash flows.
Net Present Value (NPV): Helping You Make an Investment Decision
When it comes to purchasing a new piece of equipment, office space, or any other long-term asset, it can require a big investment. And it’s also a big decision; after all, you could be spending possibly tens or hundreds of thousands of dollars.
A npv calculation can help you make an informed decision by telling you if you can expect to get a positive return on your investment.
As described here, the calculation looks at the projected cash flows of the asset, finds the present value of those cash flows, and then subtracts the cost of the initial investment to determine the net present value. If the net present value is positive, you could be looking at a good investment. If it’s negative, you may want to reconsider, because investing in the asset could cause you to lose money.
As a small business owner, you’re an expert, too. We want to hear about how you feel about discounted cash flow. Let us—and your fellow SBOs—know by sharing a comment below.
Next Steps: Want to get more tips on running your business? Sign up for the Small Biz Ahead newsletter to receive a weekly roundup of the latest tools, trends and resources.






Very informative.